Skip to main content

Project Euler Problem 67 Solution with Python

Maximum path sum II

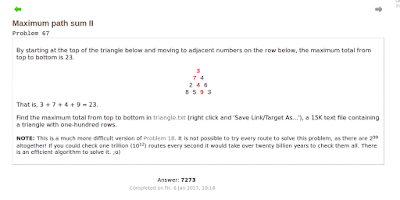
By starting at the top of the triangle below and moving to adjacent numbers on the row below, the maximum total from top to bottom is 23.
3
7 4
2 4 6
8 5 9 3
That is, 3 + 7 + 4 + 9 = 23.
Find the maximum total from top to bottom in triangle.txt (right click and 'Save Link/Target As...'), a 15K text file containing a triangle with one-hundred rows.
NOTE: This is a much more difficult version of Problem 18. It is not possible to try every route to solve this problem, as there are 299 altogether! If you could check one trillion (1012) routes every second it would take over twenty billion years to check them all. There is an efficient algorithm to solve it. ;o)

As already stated in the question, this problem is similar to problem 18. You should solve Problem 18 first and then come back here to solve this problem. 

As there are many rows in the given triangle.txt file, I have made a little bit of changes to the problem 18 python script. Unlike problem 18 solution we will open the file using the python file.read() method and everything is as usual.

Have a look at the program and it is very simple.

Program


#http://radiusofcircle.blogspot.com

#importing time module 
import time

#time at the start of execution
start = time.time()

with open('p67triangle.txt') as f:
 # All the numbers in the file
 number = f.read()

#splitting the number into a list
number = number.strip().split('\n')

#converting each and every list of strings to int
for i in xrange(1,len(number)):
 number[i] = number[i].strip().split(' ')
 number[i] = [int(x) for x in number[i]]

#adding the first number bcz we could not do the above
#operation as this one one number
number[0] = [59]

#counter for counting number of iterations
counter = 0

#for loop for bottom-up approach
for i in xrange(len(number)-2,-1,-1):
 for j in xrange(len(number[i])):
  number[i][j] = number[i][j] + max(number[i+1][j], number[i+1][j+1])
  counter += 1
 number.pop()

#printing the output
print 'Found {} in {} iterations'.format(number[0][0],counter)

#time at the end of execution
end = time.time()

#printing the total time
print end-start
You can download the script file from Github Gist 67.py

Output


Summary

As I have already solved Problem 18 using the perfect algorithm, it just took me few seconds to tweak some changes to the script file so that it can be used for this problem. Unlike 299 iterations, our program just iterates 4950 times and gives us the solution. This is less than 1% of iterations which are required when brute force method is used. So, finally I am satisfied with the program.

If you like the solution, or if you have any doubt then comment in the comment box below. I will be glad to help you.

You can also contact me.

Thank you! Have a nice day😋.
Labels:

Popular posts from this blog

Making a quiz web app with python and flask

Edit : When you are creating a web app with h tml templates, then y ou will have to sa ve the html file in templates folder in the Current Wor ki ng Directory( CWD). If you save the file in the C W D directl y you will get a TemplateNotFound error. Thank you Udhay for pointing it out.   In this post we will create a quiz website using python . I will be using the flask framework . After reading this tutorial you will learn form submission , flask templates , python code in flask templates , shuffling the questions and options with the random module and few others.  Please note that this tutorial is not big as it seems to be. Some of the code has been rewritten to maintain consistency and also font size is somewhat big so that your eyes won't get stressed reading this tutorial. Also the content has not occupied the full width of the page. In this tutorial I am assuming that you are having a very basic understanding of the flask framework . Please refer the documentation
Read more

Problem 11 Project Euler Solution with python

Largest product in a grid In the 20×20 grid below, four numbers along a diagonal line have been marked in red. 08 02 22 97 38 15 00 40 00 75 04 05 07 78 52 12 50 77 91 08 49 49 99 40 17 81 18 57 60 87 17 40 98 43 69 48 04 56 62 00 81 49 31 73 55 79 14 29 93 71 40 67 53 88 30 03 49 13 36 65 52 70 95 23 04 60 11 42 69 24 68 56 01 32 56 71 37 02 36 91 22 31 16 71 51 67 63 89 41 92 36 54 22 40 40 28 66 33 13 80 24 47 32 60 99 03 45 02 44 75 33 53 78 36 84 20 35 17 12 50 32 98 81 28 64 23 67 10 26 38 40 67 59 54 70 66 18 38 64 70 67 26 20 68 02 62 12 20 95 63 94 39 63 08 40 91 66 49 94 21 24 55 58 05 66 73 99 26 97 17 78 78 96 83 14 88 34 89 63 72 21 36 23 09 75 00 76 44 20 45 35 14 00 61 33 97 34 31 33 95 78 17 53 28 22 75 31 67 15 94 03 80 04 62 16 14 09 53 56 92 16 39 05 42 96 35 31 47 55 58 88 24 00 17 54 24 36 29 85 57 86 56 00 48 35 71 89 07 05 44 44 37 44 60 21 58 51 54 17 58 19 80 81 68 05 94 47 69 28 73 92 13 86 52 17 77 04 89 55 40 04 52 08 83 97 35 99 16 07
Read more

Problem 60 Project Euler Solution with python

Prime pair sets The primes 3, 7, 109, and 673, are quite remarkable. By taking any two primes and concatenating them in any order the result will always be prime. For example, taking 7 and 109, both 7109 and 1097 are prime. The sum of these four primes, 792, represents the lowest sum for a set of four primes with this property. Find the lowest sum for a set of five primes for which any two primes concatenate to produce another prime. This problem is j u st a brute force problem. If you have come here because you don't know the limit upto which you will h ave to gener ate the prime numbers t hen go ahe ad and t r y with 10,000 . When I first start ed solving the problem I chose 1 million(beca use most of the problem s on project E uler have this limit ), but it took very long for the computer to fin d the solution. After searching on the internet then I found many people choosing 10, 000 so I have changed my in put f rom 1 million to 10000 and the output was f ast. He
Read more